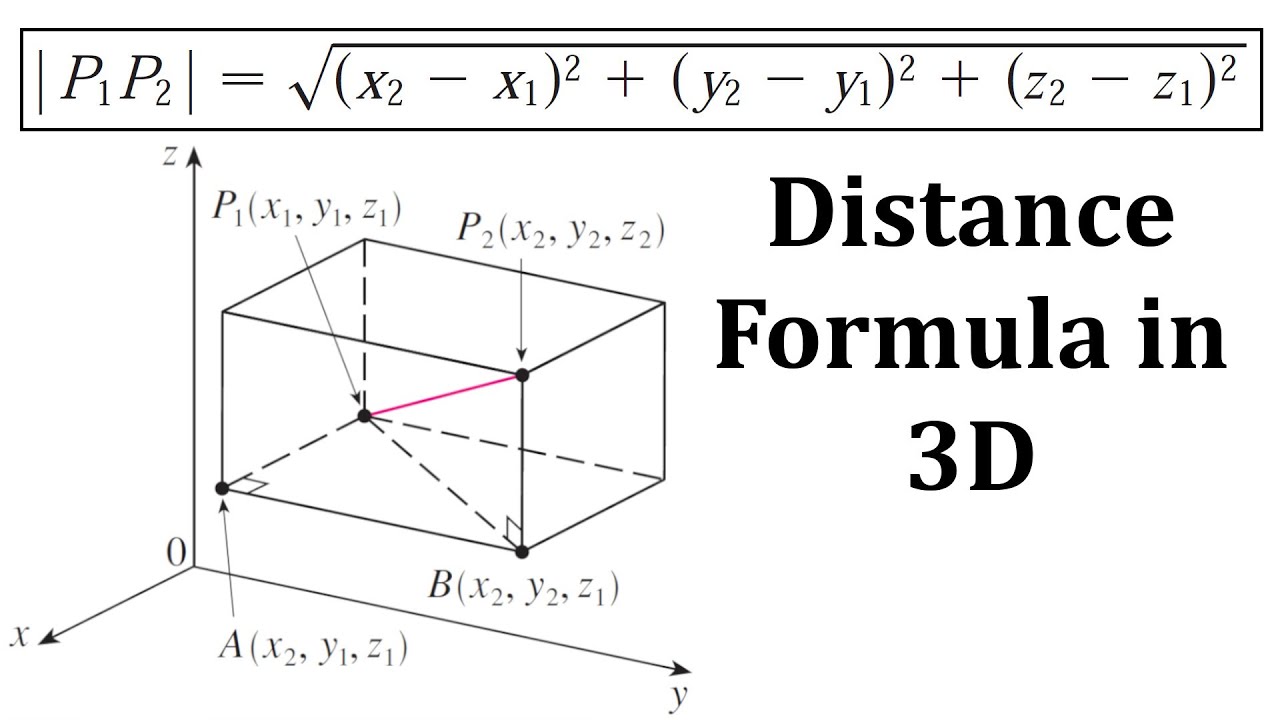
The distance formula in 3 space is a fundamental concept in geometry that helps us calculate the distance between two points in three-dimensional space. This mathematical tool is essential for various fields, including physics, engineering, computer graphics, and even in everyday applications such as navigation and architecture. In this article, we will explore the distance formula in 3 space, its derivation, applications, and examples to provide a comprehensive understanding of this important concept.
As we delve deeper into the subject, we will break down the formula, examine its components, and illustrate how to apply it in real-world scenarios. Understanding this formula is crucial for students and professionals alike who work in fields requiring spatial analysis. By the end of this article, readers will have a solid grasp of the distance formula in 3D space and its significance.
Let us embark on this mathematical journey, exploring the nuances of the distance formula in three dimensions. Whether you are a student seeking to understand geometry better or a professional needing to apply these concepts in your work, this article will equip you with the knowledge you need.
Table of Contents
- What is the Distance Formula?
- Derivation of the Distance Formula in 3D Space
- Components of the Distance Formula
- Applications of the Distance Formula
- Examples of the Distance Formula
- Common Mistakes When Using the Distance Formula
- Related Concepts in Geometry
- Conclusion
What is the Distance Formula?
The distance formula in 3D space is used to determine the straight-line distance between two points represented in three-dimensional coordinates. The formula is given as:
D = √((x2 - x1)² + (y2 - y1)² + (z2 - z1)²)
Where:
- D is the distance between the two points.
- (x1, y1, z1) and (x2, y2, z2) are the coordinates of the two points.
Derivation of the Distance Formula in 3D Space
The derivation of the distance formula in 3D space can be understood through the Pythagorean theorem. In two dimensions, the distance formula arises from the right triangle formed by the x and y differences of two points. In three dimensions, we extend this concept to include the z-coordinate.
To derive the formula, consider two points in 3D space:
- Point A (x1, y1, z1)
- Point B (x2, y2, z2)
The differences in coordinates are:
- Δx = x2 - x1
- Δy = y2 - y1
- Δz = z2 - z1
Using the Pythagorean theorem in three dimensions, the distance D can be computed as:
D = √(Δx² + Δy² + Δz²)
This leads us back to our original distance formula, reaffirming its correctness and significance.
Components of the Distance Formula
Understanding the components of the distance formula is essential for its application. The three main components are:
1. Coordinates
The coordinates of the two points in three-dimensional space are crucial. Each point is represented as (x, y, z), providing a unique location in the 3D coordinate system.
2. Differences Between Coordinates
The differences in the x, y, and z coordinates (Δx, Δy, and Δz) are calculated as the absolute differences between the respective coordinates of the two points. These differences are crucial in determining the length of the sides of the imaginary triangle formed.
3. Square Root
The square root in the formula ensures that the final distance is a non-negative value, as distance cannot be negative. It also consolidates the contributions of the differences in all three dimensions.
Applications of the Distance Formula
The distance formula in 3D space has numerous applications across various fields, including:
- Physics: Used in calculations involving forces and motion in three-dimensional space.
- Computer Graphics: Essential for rendering 3D models and animations accurately.
- Geography: Helps in determining distances between geographic locations on a three-dimensional globe.
- Engineering: Utilized in designing structures and analyzing spatial relations.
Examples of the Distance Formula
Let’s take a look at a few examples to illustrate the use of the distance formula in 3D space:
Example 1: Simple Calculation
Consider two points A(1, 2, 3) and B(4, 5, 6). To find the distance between these points, we use the formula:
D = √((4 - 1)² + (5 - 2)² + (6 - 3)²)
D = √(3² + 3² + 3²) = √27 = 3√3 ≈ 5.2
Example 2: Real-World Application
Imagine we have two cities represented by their coordinates: City X(10, 20, 0) and City Y(30, 40, 0). To find the distance between these two cities:
D = √((30 - 10)² + (40 - 20)² + (0 - 0)²)
D = √(20² + 20²) = √800 = 20√2 ≈ 28.3
Common Mistakes When Using the Distance Formula
When using the distance formula, several common mistakes can occur:
- Incorrectly identifying the coordinates of the points.
- Forgetting to square the differences before summing them.
- Neglecting to take the square root of the final sum.
Being aware of these pitfalls can help ensure accurate calculations.
Related Concepts in Geometry
Several related concepts are essential for a deeper understanding of the distance formula:
- Midpoint Formula: Used to find the midpoint between two points in 3D space.
- Vector Calculus: The distance formula is often used in conjunction with vector calculations in physics and engineering.
- Coordinate Geometry: The foundation of understanding distances, slopes, and angles in multi-dimensional spaces.
Conclusion
In summary, the distance formula in 3D space is a vital mathematical tool that allows us to calculate the straight-line distance between two points in three dimensions. By understanding the derivation, components, applications, and common mistakes associated with the formula, we can apply this knowledge effectively in various fields.
We encourage readers to practice using the distance formula through examples and to explore its applications in their respective fields. If you found this article helpful, please leave a comment below, share it with others, or check out our other articles for more insights into mathematical concepts.
Thank you for reading, and we look forward to seeing you again on our site!
Article Recommendations
- Top Wfan Radio Hosts Listen To The Best
- Sexiest Man Alive 2024 The Pinnacle Of Charm And Charisma
- Insights Into The Role And Impact Of The Wwyd Host