Understanding how to convert quadratic form to vertex form is essential for students and professionals alike, as it provides deeper insights into the behavior of quadratic equations. In this article, we will explore the step-by-step process of converting a quadratic equation from standard form, \( ax^2 + bx + c \), into vertex form, \( a(x-h)^2 + k \). Mastering this conversion is not only crucial for solving mathematical problems but also for applications in physics, engineering, and economics.
The vertex form of a quadratic equation reveals the vertex of the parabola represented by the equation, which is significant in various fields, including optimization and graphing. By the end of this article, you will have a thorough understanding of the conversion process and be able to apply it to various problems. We will provide clear examples, tips, and resources to enhance your learning experience.
So, whether you're a student preparing for an exam, a teacher looking for resources to explain this concept, or someone interested in mathematics, this article will serve as a valuable guide. Let's dive into the world of quadratic equations and learn how to convert them into vertex form!
Table of Contents
- Understanding Quadratic Equations
- The Standard Form of Quadratic Equations
- What is Vertex Form?
- Steps to Convert Quadratic Form to Vertex Form
- Example 1: Simple Conversion
- Example 2: Complex Conversion
- Common Mistakes to Avoid
- Practical Applications of Vertex Form
Understanding Quadratic Equations
Quadratic equations are polynomial equations of degree two, typically represented in the form \( ax^2 + bx + c = 0 \), where \( a \), \( b \), and \( c \) are constants and \( a \neq 0 \). These equations graph as parabolas and can open either upwards or downwards, depending on the sign of the coefficient \( a \).
Key characteristics of quadratic equations include:
- The vertex, which is the highest or lowest point of the parabola.
- The axis of symmetry, a vertical line that divides the parabola into two mirror-image halves.
- The x-intercepts and y-intercepts, which indicate where the graph intersects the axes.
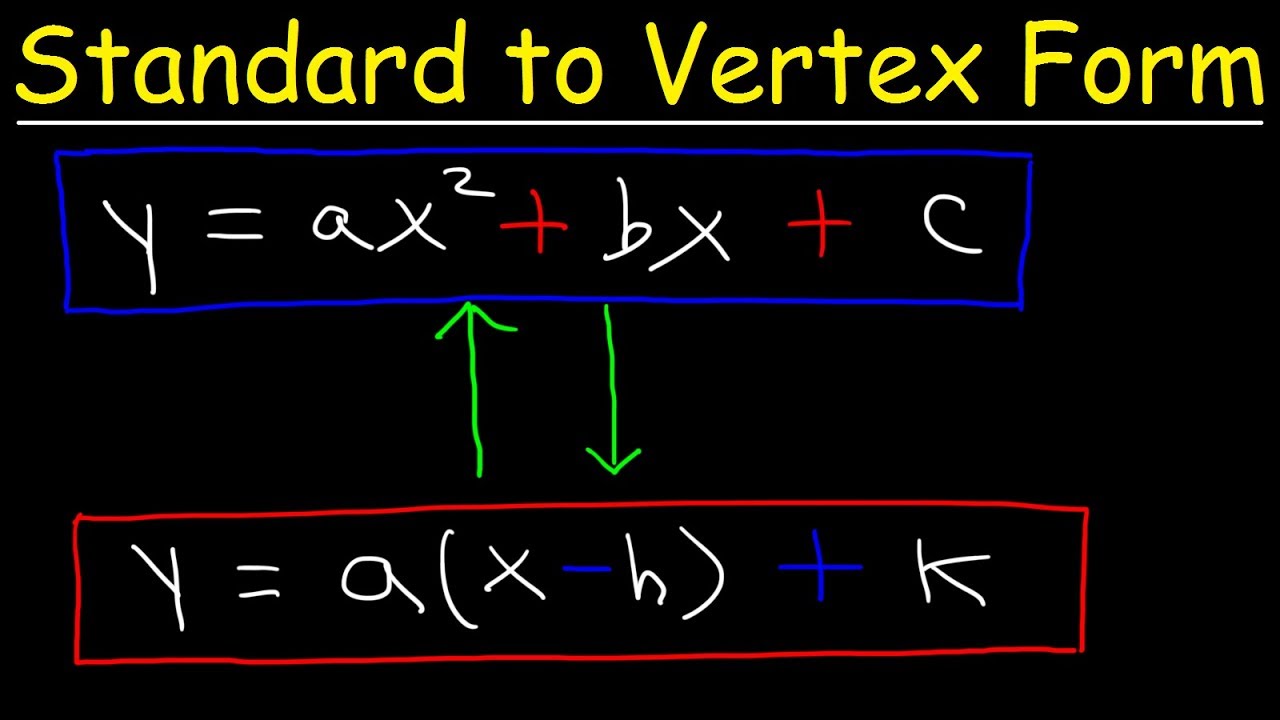
The Standard Form of Quadratic Equations
The standard form of a quadratic equation is expressed as:
f(x) = ax^2 + bx + c
In this format:
- a: The coefficient of \( x^2 \), which determines the direction and width of the parabola.
- b: The coefficient of \( x \), which influences the position of the vertex along the x-axis.
- c: The constant term, which represents the y-intercept of the graph.
What is Vertex Form?
The vertex form of a quadratic equation is given by:
f(x) = a(x-h)^2 + k
In this representation:
- (h, k): The coordinates of the vertex of the parabola.
- a: The same coefficient that indicates the direction the parabola opens.
Understanding vertex form is beneficial because it provides immediate information about the vertex and the direction of the parabola without needing to complete the square or calculate the x-intercepts.
Steps to Convert Quadratic Form to Vertex Form
Converting a quadratic equation from standard form to vertex form involves a method called "completing the square." Here are the steps:
- Start with the standard form: \( ax^2 + bx + c \).
- Factor out the coefficient \( a \) from the first two terms.
- Complete the square for the expression inside the parentheses.
- Rewrite the equation in vertex form.
Example 1: Simple Conversion
Let’s convert the quadratic equation \( y = 2x^2 + 8x + 10 \) into vertex form.
- Factor out the coefficient of \( x^2 \):
y = 2(x^2 + 4x) + 10
- Complete the square:
Add and subtract \( (4/2)^2 = 4 \):
y = 2(x^2 + 4x + 4 - 4) + 10
y = 2((x + 2)^2 - 4) + 10
- Simplify:
y = 2(x + 2)^2 - 8 + 10
y = 2(x + 2)^2 + 2
Thus, the vertex form is \( y = 2(x + 2)^2 + 2 \) with the vertex at (-2, 2).
Example 2: Complex Conversion
Now, let’s consider a more complex quadratic equation: \( y = -3x^2 + 12x - 5 \).
- Factor out the coefficient of \( x^2 \):
y = -3(x^2 - 4x) - 5
- Complete the square:
Add and subtract \( (-4/2)^2 = 4 \):
y = -3(x^2 - 4x + 4 - 4) - 5
y = -3((x - 2)^2 - 4) - 5
- Simplify:
y = -3(x - 2)^2 + 12 - 5
y = -3(x - 2)^2 + 7
Thus, the vertex form is \( y = -3(x - 2)^2 + 7 \) with the vertex at (2, 7).
Common Mistakes to Avoid
When converting quadratic equations, several mistakes may occur:
- Forgetting to factor out the coefficient \( a \).
- Incorrectly completing the square.
- Failing to simplify the final expression.
Double-checking each step can help avoid these common errors.
Practical Applications of Vertex Form
Understanding the vertex form of quadratic equations has several practical applications:
- Optimization in business and economics, where maximizing or minimizing profit or cost is crucial.
- Physics, particularly in projectile motion, where the vertex represents the maximum height.
- Graphing, as it allows for easier plotting of the parabola's vertex and axis of symmetry.
Conclusion
In conclusion, converting quadratic form to vertex form is a valuable skill that enhances your understanding of quadratic equations and their graphs. By following the steps outlined in this article, you can efficiently convert any quadratic equation and identify its vertex, which is essential for various applications. Don't hesitate to practice with different examples, and remember to avoid common mistakes.
If you found this article helpful, please leave a comment, share it with your friends, or explore other informative articles on our site!
Penutup
Thank you for reading! We hope you gained valuable insights into converting quadratic equations. We invite you to return for more educational content and resources that can help you in your mathematical journey.
Article Recommendations
- The Remarkable Journey Of Kerry Kennedys Voice Condition A Detailed Insight
- Kimberly Guilfoyles Texts Full Details Revealed
- Everything You Need To Know About Megan Foxs Halloween Party Extravaganza